High Performance Computing Facility and Computational Modelling

About 

We have developed advanced algorithms and computational models to analyse efficiently and accurately the complex ocean dynamics and their interaction with structures.

Our High Performance Computing Facility consists of:

Login node:

4x AMD Opteron(tm) Processor 6376

Each cpu has 16 cores running at 2,400 MHZ

256 GB RAM

32 TB disk memory on 7 disks running zfs with 2 redundancy disks.

Computing nodes (each):

2x Intel(R) Xeon(R) CPU E5-2697A v4 @ 3.00GHz

Each cpu has 16 physical cores.

Each core is dual threaded.

64 GB RAM

Connectivity: high bandwidth, low latency infiniBand (VPI FDR, QSFP+)

Computational models

Drag Reduction and Dynamical Systems (Dr. Tom Eaves)

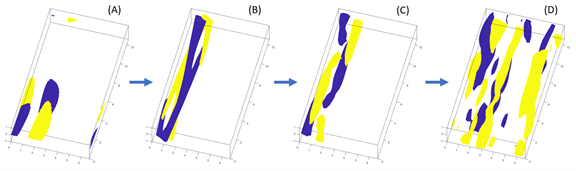
Recent observations show that substantial reductions in turbulent fluid drag can be made in turbulent boundary layers by introducing spanwise oscillation at the fluid boundary. From a dynamical systems viewpoint, it appears that the boundary oscillation excites certain invariant solutions (such as steady states or periodic orbits) which exert less drag on the boundary; the chaotic trajectory then visits these invariant solutions more frequently than it would otherwise, thus reducing the long-term turbulent drag. We are investigating the effect of periodic forcing on chaotic dynamics. The figure shows fast and slow streaks in a Couette shearing cell, starting with the smallest amplitude perturbation that can lead to turbulence(A), its evolution towards a steady-state saddle point(B), instability of this saddle point(C) and finally to fully developed turbulence(D).

Stratified shear flows (Dr. Tom Eaves)

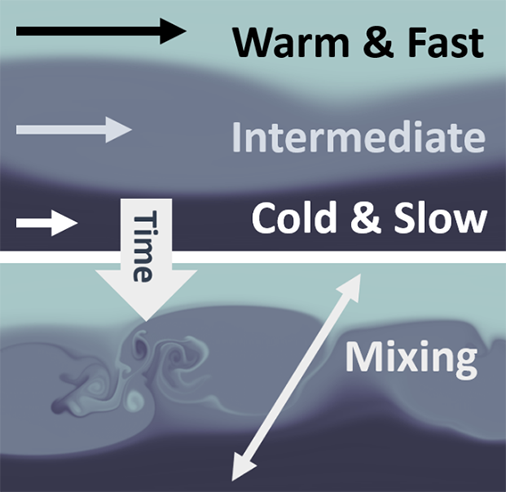
An understanding of the processes by which fluids of different densities are mixed in the ocean is crucial in order to properly constrain global climate circulation models. Local, rapid mixing processes are controlled by shear and density stratification, and such processes lie below the grid-scale resolution in climate models so that realistic parameterisation of this mixing is required. For such flows, there are three instabilities that can lead to mixing: the Kelvin–Helmholtz, Holmboe, and Taylor–Caulfield instabilities. The nonlinear evolution of each instability has distinct mixing characteristics, but it is not clear in what proportion each of these three mixing events occurs in the ocean. We use high-fidelity direct numerical simulation to help understand the dynamics of these instabilities, in order to better catalogue ocean data. The figure shows an example of the evolution of the Taylor-Caulfield instability.

Internal gravity wave-driven mixing (Dr. Anirban Guha)

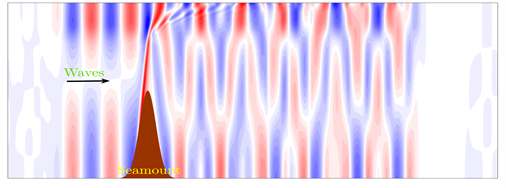
Small-scale (centimetre to the metre) mixing from oceanic internal gravity waves is essential for sustaining the Meridional overturning circulation and closing the global ocean energy budget. Weakly nonlinear wave-wave interactions and wave-(submarine)topography interactions are two of the primary mechanisms through which internal gravity waves' energy cascades to small length scales, thus leading to turbulence and mixing. We use high-resolution computational fluid dynamics simulations to study wave-wave and wave-topography interactions in realistic oceanic scenarios. The figure shows an internal gravity wave-topography interaction in the presence of a steady surface current.

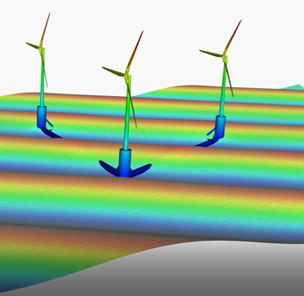
Wave-current-wind interaction with floating offshore wind turbines (Dr. Masoud Hayatdavoodi)

Floating offshore wind turbines are typically under the action of three types of external loads, namely (i) hydrodynamic loads due to waves and current, (ii) aerodynamic loads due to wind, and (iii) restoring loads due to the mooring lines attached to the seabed. Responses of structures to these environmental loads include (i) translational and rotational motions of the floating body, and (ii) the elastic deformations. We have developed computational models to determine coupled wave-wind-current loads on floating structures and analyse their motion and elastic responses.